
Interview with
Warwick’s Professor Martin Hairer
Winner of the highly
prestigious Fields Medal for Mathematics
Dr Martine J. Barons*
and Dr Paul I. Chleboun
Department
of Statistics, Zeeman Building, University of Warwick, Coventry, UK
*Correspondence:
martine.barons@warwick.ac.uk
Abstract Professor Martin Hairer was one of
four recipients of the 2014 Fields Medal, widely viewed as the highest honour a
mathematician can receive. He is currently Regius Professor of Mathematics in
the Mathematics Department at the University of Warwick. Professor Hairer has
contributed significantly to the field of stochastic partial differential equations
(SPDEs), which engages with interdisciplinary approaches to mathematics and
physics. He has enjoyed great success communicating mathematics to a range of
audiences and has also developed music editing software.
In this interview, early
career mathematicians, Dr Martine Barons (MJB) and Dr Paul Chleboun ask
Professor Hairer (MH) about how his interest in mathematics developed; the
awards ceremony where he received the Fields Medal; Amadeus Pro, the music
software he developed and continues to maintain; and the challenges of engaging
a sceptical and sometimes critical public as a mathematician.
Keywords: Martin Hairer; Fields Medal; mathematics; SPDEs; probability;
Ising universality model
Introduction
Warwick's
Professor Martin Hairer was one of four recipients of the 2014 Fields Medal,
widely viewed as the highest honour a mathematician can receive. Often
considered to be mathematics' equivalent to the Nobel Prize, it is given every
four years, and several can be awarded at once. The other recipients in 2014
were Maryam Mirzakhani, a professor at Stanford, Artur Avila of the National
Institute of Pure and Applied Mathematics in Brazil and the National Center for
Scientific Research in France, and Manjul Bhargava of Princeton University.
Professor Martin Hairer ‘has been regarded since his late 20s as a leading
figure in stochastic analysis, the branch of mathematics dealing with random
processes like crystal growth and the spread of water in a napkin. Hairer's
colleagues particularly note his rare mathematical intuition, an ability to
sense the way toward grand solutions and beautiful proofs’ (Wolchover, 2014). The theory has been
described as providing ‘both the tools and the instruction manual for solving a
huge class of previously unfathomable equations, statements that amount to
“basically, infinity equals infinity” … but which, despite their seeming
senselessness, arise frequently in physics. The equations are mathematical
abstractions of growth, the hustle and bustle of elementary particles and other
“stochastic processes”, which evolve amid environmental noise’ (Wolchover, 2014).
Martin’s
recent work on extending the theory of stochastic partial differential
equations (SPDEs) marked a significant breakthrough in the field. SPDEs describe
the large scale dynamical behaviour of random processes, such as stock market
fluctuations.
‘Without mathematics there would be
no smart phones, MRI scanners, new medicines, aeroplanes or bank accounts’ (EPSRC & Council for the Mathematical
Sciences, 2012: 3). One report commissioned by the Engineering and Physical
Sciences Research Council (EPSRC) and the Council for the Mathematical Sciences
(CMS) has calculated that ‘10 per cent of jobs and 16 per cent of Gross Value
Added (GVA) to the UK economy stems from mathematical sciences research’ (EPSRC & Council for the Mathematical
Sciences, 2012: 2). It was therefore a great privilege and most enjoyable
to interview Professor Martin Hairer, who has brought so much prestige to the
University of Warwick through his achievements and the high profile Fields
Medal. It is a great gift to be able to communicate, as he does, the enormous
value of mathematical research of all kinds to such a broad range of audiences.

The Interview
MJB: What drew you into mathematics and into
stochastic partial differential equations (SPDEs) in particular?
MH: I was always interested in
science and I did some programming as a teenager, so I did quite a lot of
computing and also took part in science competitions for High School students with
some computer software. Then it was difficult to choose between mathematics,
physics and computer science, and, in the end, I studied physics. My father is
a mathematician, so I certainly had some exposure to mathematics from an early
age and was always interested, but chose physics partly to establish my own
identity. Within physics, I was always more interested in the theoretical side
than the experimental side. On the theoretical side, I was much more
comfortable with mathematics because you could prove what you were claiming,
whereas in physics you could have an argument for it but it wouldn't be
definite proof. So I felt that, if I were to write a paper and put my name to
it, I would want it to actually be correct for sure. In mathematics I could
have that assurance that if I proved something it was actually true and it's
true for ever.
MJB: And what about SPDEs in particular? What's the main attraction
there?
MH: So that's actually the link back
to physics. Within mathematics I was still always interested in problems or
subjects that do have something to do with the ‘real world’, because I find it
much easier to build an intuition on the analytical side of mathematics than on
the more algebraic or combinatorial side.
Martin revealed that he tends to work from an intuition and then go to
the algebra to describe the intuition to see if his intuition is really
correct.
MJB: Can you give a non-mathematician's introduction to what you do and
why you do it?
MH: I'll try! I can try to explain
some of the things I've been doing recently, for which I was awarded the Fields
medal. In a nutshell, what I was trying to do was to give a consistent
interpretation to some equations that, on the face of it, shouldn't actually
make any sense. One example is the equation that describes the fluctuations of
an interface. For example, if you take a piece of paper that starts burning, at
the beginning the interface is smooth, but then some bits burn a bit faster
than others, so it starts wobbling and becomes more and more wobbly (Figure 2), and then you are interested
in describing this process at very large scales. So you zoom out at very large
scales, imagine a kilometre-long sheet of paper and you adjust the vertical
zoom factor so that you see something moving. What you actually end up seeing
is something extremely rough and when you try to get to the idealised object from
looking at infinitely large scales, you see something infinitely rough. The
equation that is supposed to describe the evolution of that object involves the
square of the derivative (the slope at each point).
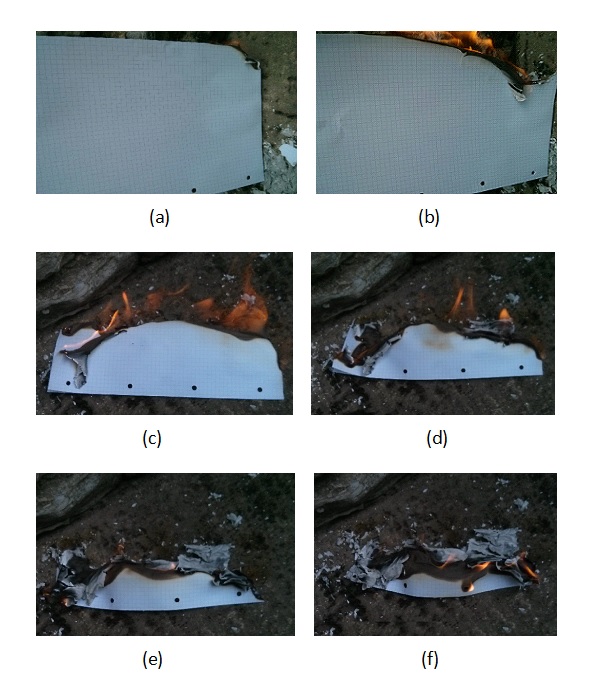
Now the thing is so rough that it
doesn't have a slope at any point; in some sense it is infinitely steep
everywhere, it goes up and down so fast. So if you try to just take the slope
and square it, you just get infinity everywhere. So what you have to do, in
some sense, is to subtract some kind of infinitely large constant that cancels
out these infinities, but there is still something left that is not constant.
So I developed a systematic theory to treat the equations where you have these
types of phenomenon occurring, allowing you to give a mathematically rigorous
meaning to these equations and also to justify, to some extent, how they appear
in order to give a robust theory. These equations are idealised because in
reality you do not zoom out infinitely far. So you want some results to tell
you that if you have something that is approximately described by this
equation, then in certain limits it converges to this idealised object. It's a
little bit like when mathematicians talk about circles, the circle is a
completely idealised mathematical object. In the real world that (circular)
table would be described by a mathematician as a circle, but of course it is
not exactly a circle: if you take the border of the table and look in detail it
is not going to be a perfect shape, it's going to fluctuate a little, but it is
going to be extremely well described by a circle.
MJB: Receiving the Fields Medal must have been very exciting. How did you
hear the news and what was the ceremony like?
MH: I heard the news six months
before the ceremony. There had been rumours; everybody knows more or less who
has been nominated or is being considered. It is not really official knowledge,
but there are a sufficiently many rumours to get a general consensus on who is
being considered, and I was part of that general consensus. I was actually
shocked the first time somebody mentioned it to me. That was something like three
or four years ago. I was at a conference and somebody actually just asked me
‘do you think you will get the Fields medal for that work?’ I was just
completely shocked!
I heard about it officially when I
got an email from Ingrid Daubechies, who is the president of the IMU
(International Mathematical Union), asking for a phone number where she could
reach me—presumably she tried to phone me at my office, but I was a way giving
a lecture series in New York. If you get an email from Ingrid around that time
of year and you know you are being considered, then you know what is going on,
right?! Then she called me up and told me the official news, and also made sure
I knew it wasn't a joke (you want to be sure about these things). She sent out
an email with a copy of the official letter with her signature. I think Cédric
Villani (awarded the Fields Medal in 2010) mentions in his book that when the
president of the IMU called him up to tell him, he was very happy, but then
when he put down the phone suddenly thought maybe it's a joke!
MJB: Where was the ceremony and what was it like?
MH: The ceremony was in Seoul in
South Korea last summer. It was big: there were probably about five thousand
people in the hall. It was chaired by the South Korean president so therefore
all five thousand attendees had to go through airport-style security to get
into the hall. Those of us who got close to her—the four medal winners and a
few others—had a rehearsal with the security brief the evening before, and were
given very precise instructions on what we were allowed to do and what we were
not allowed to do: ‘at this moment move, take two steps forward, but don't move
too far forward; leave enough time for the security guys in the room to
reshuffle themselves, don't make any sudden movements or else you will make
them very nervous.’ It was kind of fun and an interesting experience!
At the opening ceremony, they made a
big thing about the award with movie presentations and so on. They had made a three-minute
movie for each of the four medallists, and introduced a lot of drama. They also
had lots of shows, Taekwondo demonstrations by the South Korean's top Taekwondo
club, which was really impressive, and traditional dance and traditional South
Korean music. It was really nice, a really nice ceremony. It was about two
hours long.
One of the aspects of the job that many academic mathematicians enjoy is
the acceptability of wearing casual clothes to work. Very few choose to wear a
suit, and those occasions where a suit might be considered essential are
generally few and far between. Martin told me he has one suit, but another
medallist did not own one at all and had to buy one especially for the
ceremony!
MJB: Now, you gave a very good public talk at Warwick on your technical
work. How do you approach explaining such specialist work to a general
audience?
MH: Well, you just try to put
yourself into the shoes of the audience. I am quite used to it, because I have
been asked quite a few times to give talks to a general mathematical audience,
with at least a level of undergraduate mathematics. Since I have given quite a
lot of these lectures, I now have some idea at which level to pitch it; what
you can do and what you can't do. But it takes some time to prepare these kinds
of lectures. For example, this week I gave one in Edinburgh and was briefed to
give it for a genuinely general audience, so non-mathematicians, and it
took quite a while to prepare. But people seemed to like it; it was quite well-received. But then, of
course, you go much more into historical anecdotes and these kinds of things so
people can more directly relate to it, but still find a thread of some
mathematical problem that is interesting and still relates to the things on
which people do mathematical research.
MJB: In that sort of situation do you stay with pictures and anecdotes or
do you show equations as well?
MH: I wrote down one equation at one point
on a slide without expecting people to understand it, just to show them what it
looks like.
MJB: Your work is often closely related to work in the physics community.
Do you find there is a lot of interaction between the mathematics and physics
communities within your field?
MH: Actually not so much. I know some
theoretical physicists and do have some contact with them, but I still do what
most would consider pure maths and not so close to theoretical physics. I therefore
interact much more with analysts and probabilists who do PDE theory.
MJB: Have physicists been able to use any of your results?
MH: It's not the type of result that
physicists would find useful in that sense—it's more about giving a different
and deeper understanding of things that they've been saying. In some sense, it
vindicates some of what physicists have been saying, so there is some
cross-over in that sense.
MJB: You also produced some music editing software. How did you become
interested in this, and how is it related to your mathematical work?
MH: I started doing that ages ago
when I was still in High School; it was a project I developed for one of these
High School science competitions. The original plan was to write a piece of
software that would take a recording and then be able to extract the musical
score from the recording. It turned out to be a bit over-ambitious! So I did
part zero of the project, which is to actually get the recording into the
computer and being able to manipulate it and do something with it. And then,
for a while, it was just an interesting side project I had. One goal was to use
it to learn programming properly, so this became my test project. But then it
became quite successful. There's not much of a direct link to mathematics. Of
course it helps to know mathematics, such as the Fourier transform; for the
basics of signal processing you need to know some mathematics, so it is certainly
helpful in that sense. But, on the other hand, I started working on this before
I had even started studying mathematics.
Amadeus Pro is the software that Martin has developed. In contrast to programmes
like Cubase, which started as a midi editor, Amadeus is a wave editor
supporting a variety of formats. Amadeus Pro is commercially available at: http://www.hairersoft.com/pro.html
MJB: Are you still keeping Amadeus Pro updated? Is it still being downloaded?
MH: Oh yes, of course. I don't have
that much time to spend on it, but I still maintain it sufficiently so it
continues to run smoothly on the latest operating system. Every time they
release a new operating system, there are always small glitches. This could
take anything from a day to a week. What takes much more time is providing some
level of customer support. I get emails from people who use it and I have to
reply to these emails. This takes maybe half an hour per day and I usually have
of the order of six or seven emails a day to which I have to reply. Turnaround
depends on where I am and what I'm doing, but is usually quite fast.
MJB: That's very admirable: you are producing new mathematics and still
have really excellent customer service! How do you approach your work and come
up with ideas? What inspires you and how do you like to work? You said
something already about how originally you were in physics and you had some
ideas for there. What about your sources of inspiration more generally?
MH: In general, it's difficult to
say. Sometimes you know what the interesting problems in an area are, because
you go to conferences and you talk to people. Usually people have a fairly good
picture given the area that they work in and the problems that are of interest
to other people.
How do I like to work? Well, it
depends on which part of working. There is the bit that involves actually figuring
out a new technique to deal with a type of problems, and there is the part of
actually working out the details and making sure that it works. And then there
is the writing up, which takes quite a lot of time. The second and third parts
are for me very much related in the sense that I often start writing things up
before I have worked out all the details, so I work out details as I write.
MJB: So it's an iterative process; the need to write it down drives the
need to get the details from the big picture?
MH: Yes. Sometimes I find it's only
when I really start to write things down that I realise that I have overlooked
a certain aspect or case. When you start
to actually write things down and explain everything with details you realise
actually, I haven't thought about that!
MJB: Having embarked on this field, do you know where the search
directions are that you would like to pursue to some degree?
MH: Yes. Since I have developed this
whole theory that is very new, I have quite a lot of projects going on at the
moment. I am working out details and extending them to other types of problems
and so on, so that's going to keep me busy for a bit!
MJB: How do you see this branch of mathematics developing?
MH: Well, there are certainly some
big open problems that people are aware of and are trying to work towards.
There's one thing that is somehow very badly understood and is very important
in probability theory, at least the kind of probability theory in which I am
interested (the one that relates to physics). There's this notion of
universality that says that large-scale behaviour of a system doesn't really
depend too much on the details of how it is made up at smaller scales. People
have some kind of intuitive understanding of why that's true and some heuristics
of what these different universality classes are. But there's actually very
little that is known in a rigorous, mathematical sense. We know things about
those classes where we can compute things and have explicit formulae: we know a
formula for the Gaussian distribution, so we have a really good understanding
of the scope of the central limit theorem, we know that it's more than sums of
iid random variables. There is a kind of intuitive understanding of roughly
what ingredients it takes to have a central limit theorem and then you have
lots of tools to actually do it and it helps to have an actual formula for the
Gaussian distribution. Now, there are other examples of these universality
classes and for some of them one doesn't have a formula, so there is still very
little that is known rigorously. One example is the fluctuations of a magnet:
if you take a magnet, which has a magnetic field, and heat it up at a critical
temperature, it loses its magnetic field. And now you are interested in what
happens exactly at this critical point: inside the magnet the field fluctuates
very wildly and there is a universality class associated with this and there
is, in principle, a scaling exponent associated to this, but one doesn't even
know the exponent—the thing that would be ½ for the central limit theorem! It's
not just that you don't know any formula for anything, but you don't even know
the correct way to rescale it when you look at large scales. Trying to
understand that is a pretty good source of problems.
MJB: So what is the particular universality class called?
MH: It is the Ising universality
class because of the Ising model. In two dimensions it is relatively well
understood because of conformal symmetries and so on, but in three dimensions
it is not known. Then in higher dimensions again it becomes trivial at some
point; actually, it becomes Gaussian at some point.
The Ising model, named
after the physicist Ernst Ising, is a mathematical model of magnetic materials.
The material is modelled as a discrete structure, such as a grid, with +1 or -1 on each vertex, representing the local orientation of the magnetic
field. Mathematicians are interested in the macroscopic (overall) magnetic
properties of the material given by the average over the microscopic magnetisation
at each vertex, much as sociologists are interested in how the actions of
individuals impact on society as a whole.
MJB: So there's some
interesting middle ground, here; if it's very large, you know what you're doing
and, if it's very compact you know what you're doing, but in the middle it's
interesting.
MH: Yes, somehow one and two dimensions tend to be special and you know
what to do. Then in three dimensions nobody has a clue about anything. Starting
from four dimensions it becomes easier again, just a Gaussian behaviour. We
just live in three dimensions!
MJB: So this is the most difficult dimension to live in?
MH: Well, I suppose it's the most
interesting one!
MJB: Yes, that's a more positive way to look at it! If you were advising
a young person who was wondering whether to do mathematics, wondering if it is
really interesting or is it just sitting in a corner doing nothing important,
how would you advise them?
MH: Well, first of all it depends
very much on the person's personal tastes, for example if they actually have an
interest in mathematics. If they like mathematics, they should definitely go
for it and there are definitely still things to explore and lots of things to
do; it is certainly not a finished, dead field that was worked out in the
nineteenth century!
MJB: How do you cope with the problem that a lot of people don't really
understand what mathematicians do and so when you say, ‘I'm a mathematician’
they say ‘oh’ or ‘I was rubbish at mathematics at school’?
MH: I think one can try to explain to
people what mathematicians do at some kind of high level without going into
details. One thing is that people tend to be scared of equations, of
mathematical formulae. Of course, at the end of the day, the only reason that
we use formulae is because it is a compact way of describing something very
precisely. We could turn every equation into a piece of text, it's just that
the one-line equation might be half a page of text or more, so it is easier to
visually take in one line than half a page, therefore it is much more efficient
to communicate using these equations. If you wrote out all the text there would
be so much clutter, and it would be much more difficult to actually make sense
of what is being said. Of course, it does depend on being able to speak or read
the language, but then that's just like any foreign language; if you see
something written in Chinese it doesn't make any more sense than a bunch of
maths formulae, but if you learn Chinese...
Conclusion
As discussed during the interview,
Professor Hairer’s work is closely related to understanding models of huge
importance to the physics community. Mathematicians are increasingly
collaborating with biologists, chemists, sociologists, and archaeologists among
others, in order to provide new and deeper insights previously out-of-reach in
these fields. On 15 May 2015, for example, Professor Hairer spoke at an event
hosted by the Institute of Advanced Study (University of Warwick) on ‘The
future of Interdisciplinary Research’ at The Shard, London.
There is a need to engage the wider
public with academic activity and enhance understanding of the value of our
efforts to society as a whole. This can be particularly difficult in highly
technical domains such as mathematics, but with ingenuity and effort, can be
achieved. A number of high profile mathematicians, including Martin Hairer, are
making significant efforts to engage with the public. This interview has shown
the importance of Professor Hairer’s research for engaging a broad academic and
non-academic community, as well as his ability to convey complex, high-level
information in a way that is interesting and engaging.
About the Authors
Paul Chleboun and Martine Barons both took their MSc and PhD studies at
the University of Warwick’s Complexity Science Doctoral Training Centre. Paul’s
thesis was on Large Deviations and Metastability in Condensing Stochastic
Particle Systems. He moved on to postdoctoral work in Rome before returning to
Warwick as an IAS Global Research Fellow in January 2013. Martine’s thesis was
titled ‘What is the added value of using non-linear models to explore complex
healthcare datasets?’ She has since worked as a Research Fellow in Warwick’s
Department of Statistics on the subject of methodology development and the application
of graphical models for decision support in complex environments.
References
EPSRC & Council for the Mathematical Sciences (2012).
‘Mathematical Sciences Research: Leading the Way to UK Economic Growth’: http://www.ima.org.uk/_db/_documents/4_page_economic_impact.pdf [accessed on 4 September 2015]
Wolchover, Natalie (2014). ‘In noisy equations, one who heard music’, Quanta Magazine: https://www.quantamagazine.org/20140812-in-mathematical-noise-one-who-heard-music/ [accessed on 4 September 2015]
Barons, M. J. & Chleboun, P. I. (2014), ‘Interview with
Warwick’s Professor Martin Hairer’, Exchanges:
The Warwick Research Journal, 3(1), 12–23. Retrieved from: http://exchanges.warwick.ac.uk/index.php/exchanges/article/view/79